Introducing the quiz on parallel lines and transversals! Delve into the captivating world of geometry as we explore the fundamental concepts, properties, and applications of these intriguing lines. Brace yourself for a journey that will illuminate your understanding of parallel lines and transversals, empowering you to solve complex problems and unravel the mysteries of the geometric realm.
Parallel lines, like steadfast companions, maintain an unwavering distance from each other, never intersecting. Transversals, on the other hand, play the role of intermediaries, crossing parallel lines and revealing their hidden relationships. Together, they form a geometric tapestry that unveils a symphony of angles and properties.
Defining Parallel Lines and Transversals: Quiz On Parallel Lines And Transversals
Parallel lines are two lines that lie in the same plane and never intersect. They maintain a constant distance from each other throughout their entire length. Transversals are lines that intersect two or more other lines. When a transversal intersects two parallel lines, it creates eight distinct angles.
Properties of Parallel Lines and Transversals
- If two lines are parallel, then the corresponding angles formed by a transversal are congruent.
- If two lines are parallel, then the alternate interior angles formed by a transversal are congruent.
- If two lines are parallel, then the alternate exterior angles formed by a transversal are congruent.
- If two lines are parallel, then the vertical angles formed by a transversal are congruent.
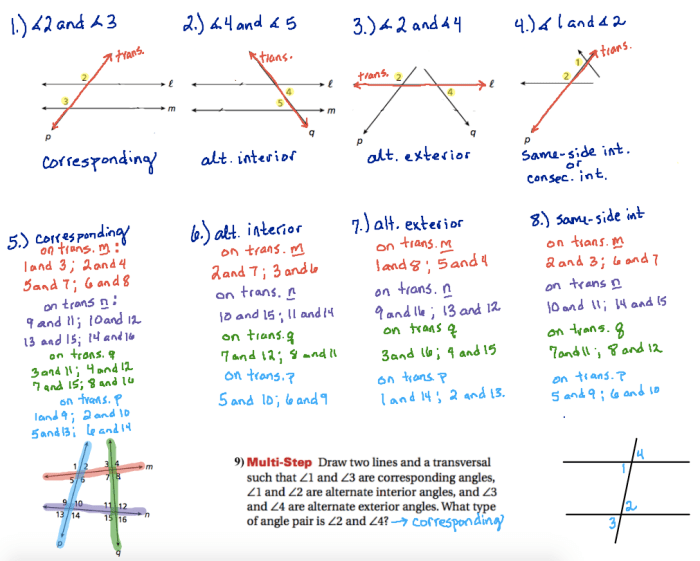
Angle Relationships Formed by Transversals
- Corresponding angles: These are angles that are in the same position relative to the parallel lines and the transversal. They are congruent if the lines are parallel.
- Alternate interior angles: These are angles that are on opposite sides of the transversal and inside the parallel lines. They are congruent if the lines are parallel.
- Alternate exterior angles: These are angles that are on opposite sides of the transversal and outside the parallel lines. They are congruent if the lines are parallel.
- Vertical angles: These are angles that are opposite each other and formed by the intersection of two lines. They are always congruent.
Solving Problems Involving Parallel Lines and Transversals
To solve problems involving parallel lines and transversals, follow these steps:
- Identify the parallel lines and the transversal.
- Determine the type of angles that are being formed.
- Use the properties of parallel lines and transversals to solve for the unknown angles.
Applications of Parallel Lines and Transversals, Quiz on parallel lines and transversals
Parallel lines and transversals have many practical applications in fields such as architecture, engineering, and surveying. For example, architects use parallel lines to create the framework of buildings, and engineers use transversals to calculate the angles of bridges and other structures.
FAQ Section
What are the key properties of parallel lines cut by a transversal?
Parallel lines cut by a transversal form eight distinct properties, including alternate interior angles being congruent, corresponding angles being congruent, and vertical angles being congruent.
How can we use parallel lines and transversals to solve real-world problems?
Parallel lines and transversals find practical applications in various fields. For instance, architects use them to design buildings with parallel walls and perpendicular floors, while engineers employ them to construct bridges with parallel beams and evenly distributed loads.
What are the different types of angles formed when a transversal intersects parallel lines?
When a transversal intersects parallel lines, it forms four types of angles: alternate interior angles, corresponding angles, alternate exterior angles, and vertical angles.